A light rod of length ( has two masses m, and me attached to its
Por um escritor misterioso
Last updated 12 abril 2025

Click here:point_up_2:to get an answer to your question :writing_hand:a light rod of length has two masses m and meattached to its two
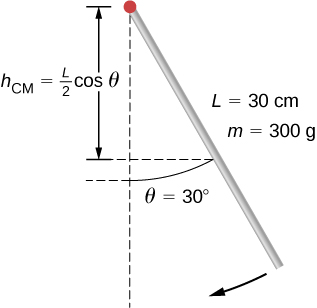
10.5 Calculating Moments of Inertia
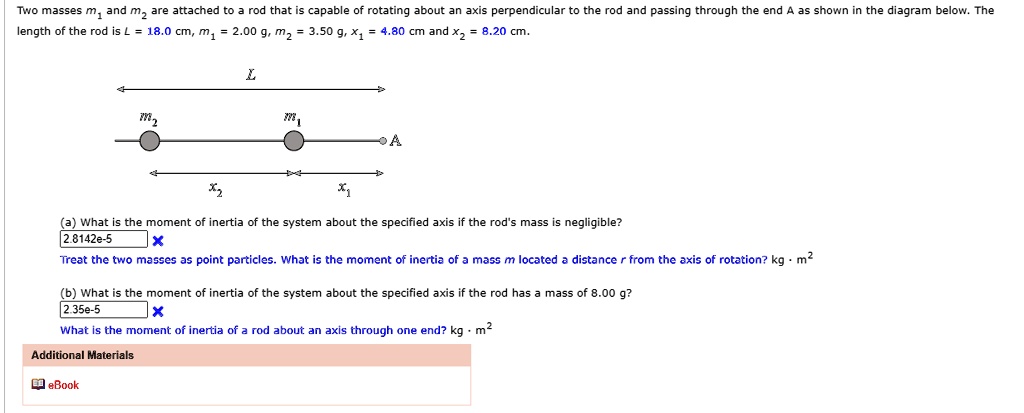
SOLVED: Two masses are attached to a rod that is capable of rotating about an axis perpendicular to the rod and passing through one end, as shown in the diagram below. The
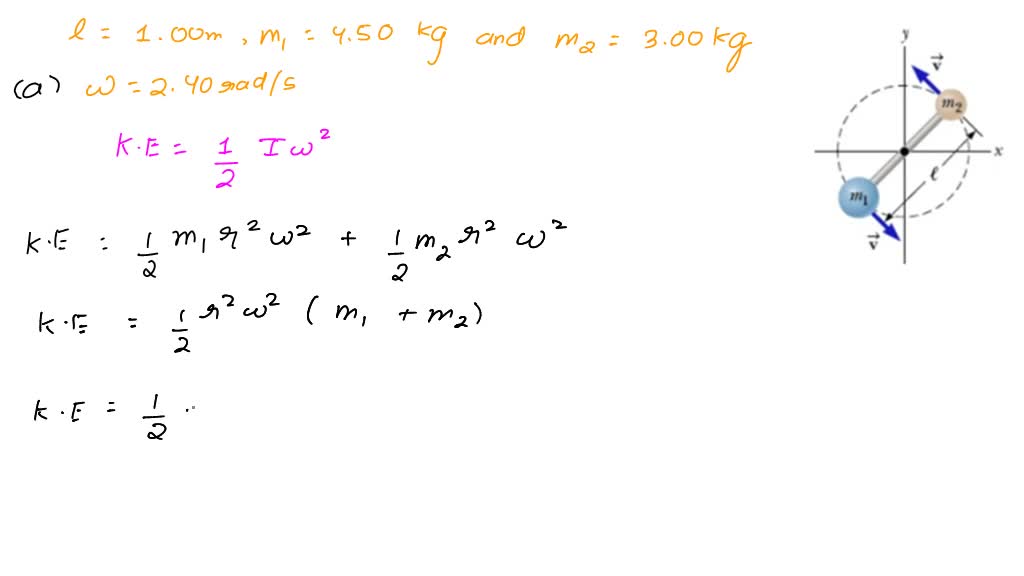
SOLVED: light rod of length 1.00 m rotates about an axis perpendicular to its length and passing through its center as in the figure below. Two particles of masses m1 4.50 kg

Lagrangian mechanics formulation of a simple free motion of two masses in uniform gravity field - Physics Stack Exchange

homework and exercises - Lagrangian function for two swivelling masses attached by a spring - Physics Stack Exchange

ANSWERED] One end of an ideal spring is fixed at point O and o - Physics - Kunduz
Two masses are tied together and suspended over a frictionless pulley. The masses are 10.0 kg and 15.0kg respectively. The blocks are released from rest. Find the acceleration of the blocks? What
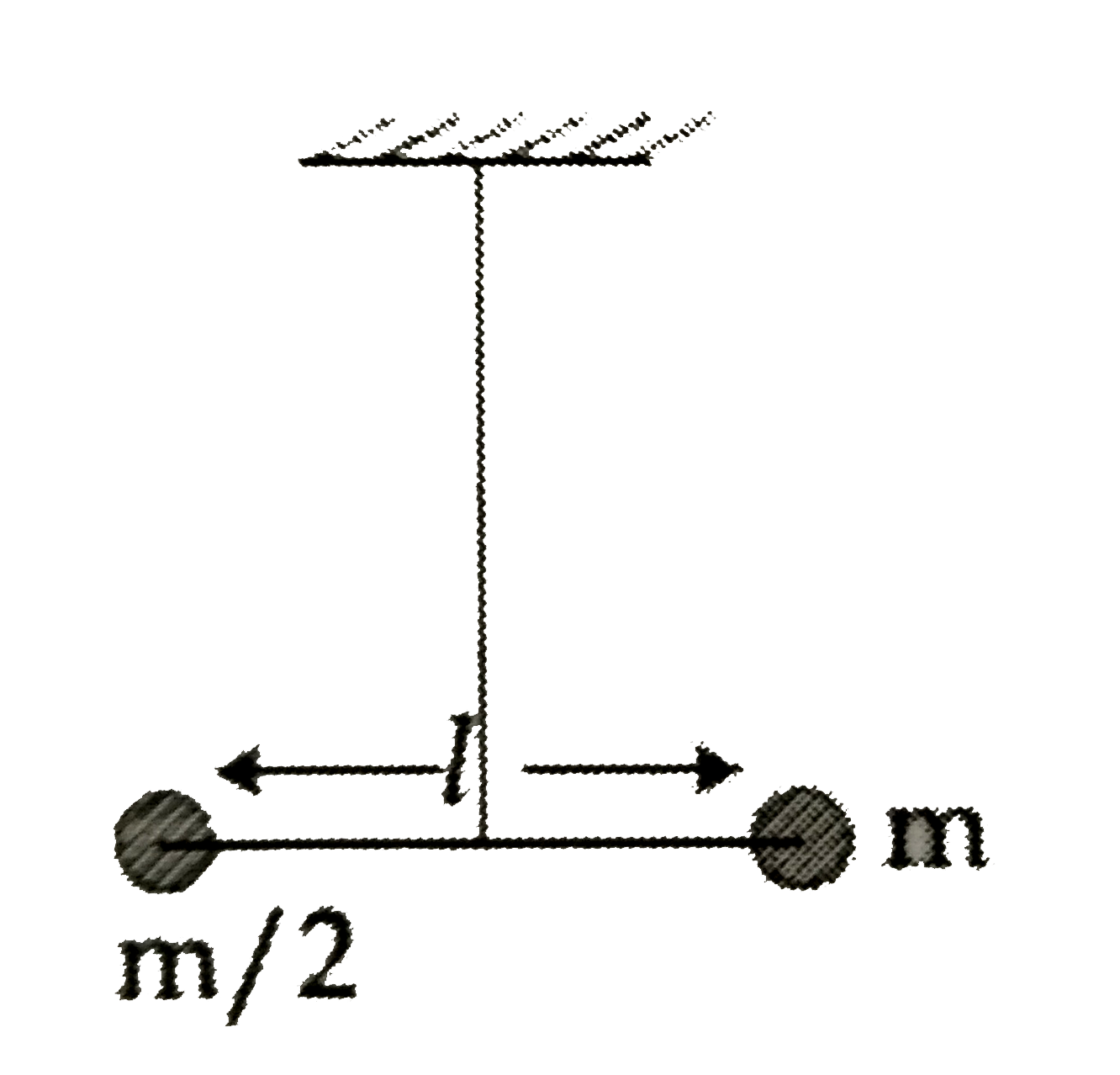
Two masses m and (m)/(2) are connected at the two ends of a massless r
23. Two point masses m are connected to the light rod of length I and it is free to rotate in vertical planeas shown in figure. Then the minimum horizontal velocity that
Recomendado para você
-
 Vollgewindestange Gewindestange Doppelkopfschraube Edelstahl 304 MM2 M2,5 M312 abril 2025
Vollgewindestange Gewindestange Doppelkopfschraube Edelstahl 304 MM2 M2,5 M312 abril 2025 -
 freeshipping 0.1mm2-16mm2 M0 M1 M2 M3 Arabic Numerals M Type Clip Network Ethernet Wire Number Label Tube Cable Marker colorful - AliExpress12 abril 2025
freeshipping 0.1mm2-16mm2 M0 M1 M2 M3 Arabic Numerals M Type Clip Network Ethernet Wire Number Label Tube Cable Marker colorful - AliExpress12 abril 2025 -
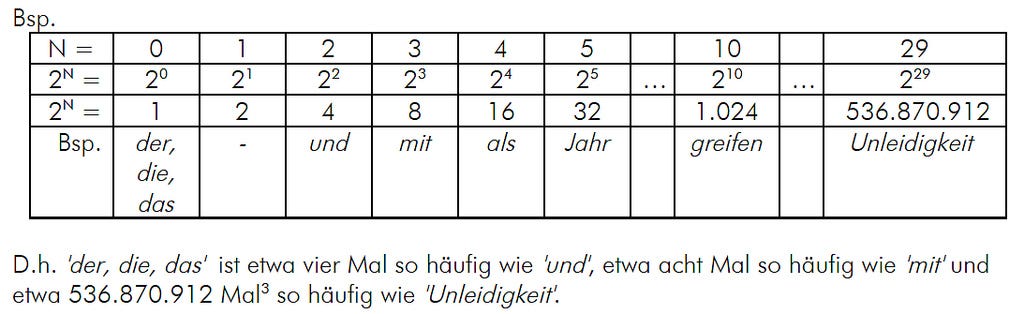 Comparing Lexical Frequency. Searching for a Meaningful Buckets, by David Rosson, Linguistic Curiosities12 abril 2025
Comparing Lexical Frequency. Searching for a Meaningful Buckets, by David Rosson, Linguistic Curiosities12 abril 2025 -
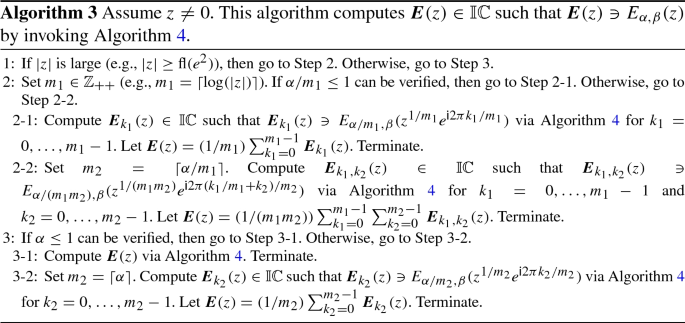 Computing Enclosures for the Matrix Mittag–Leffler Function12 abril 2025
Computing Enclosures for the Matrix Mittag–Leffler Function12 abril 2025 -
 Chuyển đổi Milimet vuông (mm2) sang Mét vuông (m2)12 abril 2025
Chuyển đổi Milimet vuông (mm2) sang Mét vuông (m2)12 abril 2025 -
 39. A stress of 1.5 kg.wt/mm2 is applied to a wire of Young's12 abril 2025
39. A stress of 1.5 kg.wt/mm2 is applied to a wire of Young's12 abril 2025 -
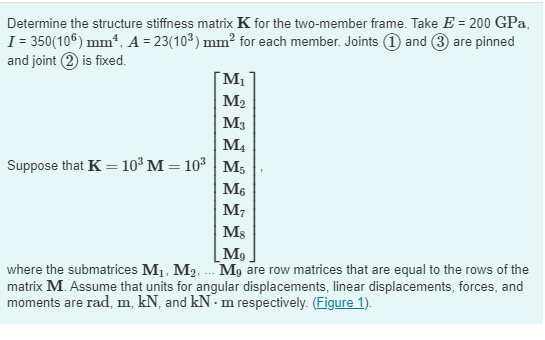
Solved Determine the structure stiffness matrix K for the12 abril 2025
-
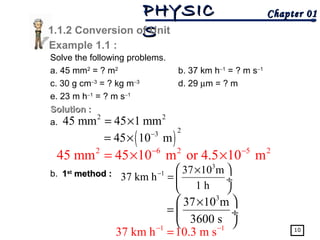 1.0 Physical Quantities and Measurement12 abril 2025
1.0 Physical Quantities and Measurement12 abril 2025 -
 Measures and Units 2 Areas and Volumes mm 2 km 2 m3 ? cm 2 mm 2 cm12 abril 2025
Measures and Units 2 Areas and Volumes mm 2 km 2 m3 ? cm 2 mm 2 cm12 abril 2025 -
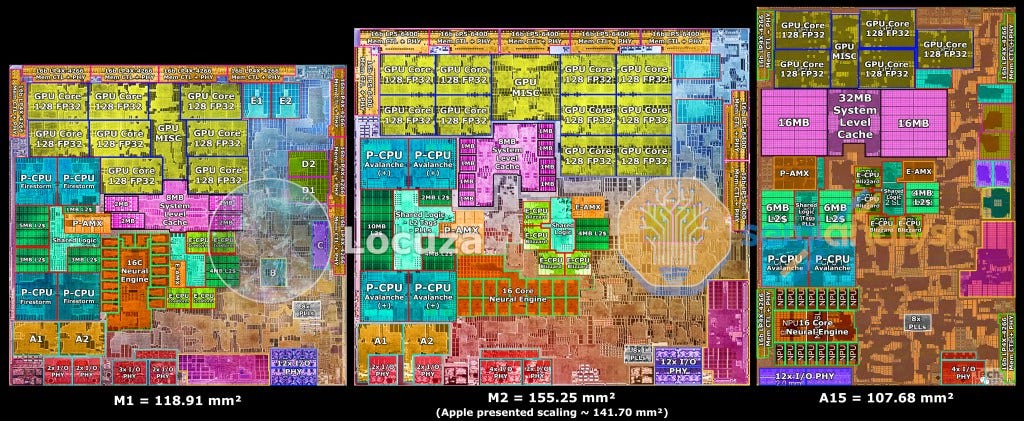 Apple M2 Die Shot and Architecture Analysis – Big Cost Increase12 abril 2025
Apple M2 Die Shot and Architecture Analysis – Big Cost Increase12 abril 2025
você pode gostar
-
 Dance Charts Step 412 abril 2025
Dance Charts Step 412 abril 2025 -
Final Boss assistance - spoilers within - Metal Gear Rising12 abril 2025
-
 Resultados da pesquisa por “booth” – Chair & Table Blog – Cadeiras e Mesas12 abril 2025
Resultados da pesquisa por “booth” – Chair & Table Blog – Cadeiras e Mesas12 abril 2025 -
 Europa-Park extends its partnership with Racing Club de Strasbourg Alsace until 2025 - Racing Club de Strasbourg Alsace12 abril 2025
Europa-Park extends its partnership with Racing Club de Strasbourg Alsace until 2025 - Racing Club de Strasbourg Alsace12 abril 2025 -
![FILME] Dragon Ball Z: A Galaxia Esta em Perigo, Universo do Dragão](https://sun9-80.userapi.com/impf/c636222/v636222727/2b71d/qFo4aUqX0JQ.jpg?size=271x186&quality=96&sign=9a7d7a8b0b84ae8d7dde0fea564a13f2&type=album) FILME] Dragon Ball Z: A Galaxia Esta em Perigo, Universo do Dragão12 abril 2025
FILME] Dragon Ball Z: A Galaxia Esta em Perigo, Universo do Dragão12 abril 2025 -
 2023 World Chess Championship: Who will follow Carlsen? – DW – 04/06/202312 abril 2025
2023 World Chess Championship: Who will follow Carlsen? – DW – 04/06/202312 abril 2025 -
 Especial Mortal Kombat #4: As inúmeras realidades alternativas12 abril 2025
Especial Mortal Kombat #4: As inúmeras realidades alternativas12 abril 2025 -
 Ampere Analysis Insights12 abril 2025
Ampere Analysis Insights12 abril 2025 -
 tropa do calvo12 abril 2025
tropa do calvo12 abril 2025 -
 Plants vs. Zombies 2 Gameplay Walkthrough - Episode 21 - Far Future! Laser Bean! Citron!12 abril 2025
Plants vs. Zombies 2 Gameplay Walkthrough - Episode 21 - Far Future! Laser Bean! Citron!12 abril 2025